Math is perennial; we should better understand the fathers of math for their approaches were of ageless beauty and simplicity.
Math is perennial and a good way to teach my students is to ponder with them on the problems that surround us, just as our Founding Fathers of Mathematics once did. As an English teacher believes students must read Dickens and Shakespeare for their timelessness and superior quality – so too are students stimulated to think more deeply about math by reflecting on the classic Mathematicians and their approaches to math. Whether it be a problem (e.g. How to erect a straight wall? How many seeds are needed for a farm?) or a curiosity (What is the largest prime number? Whether there is an infinite number of twin primes?), understanding math’s perennial aspects enables the classroom to see what we are doing in the larger context. See Figure 6 for a simple example of a problem we may explore in class.
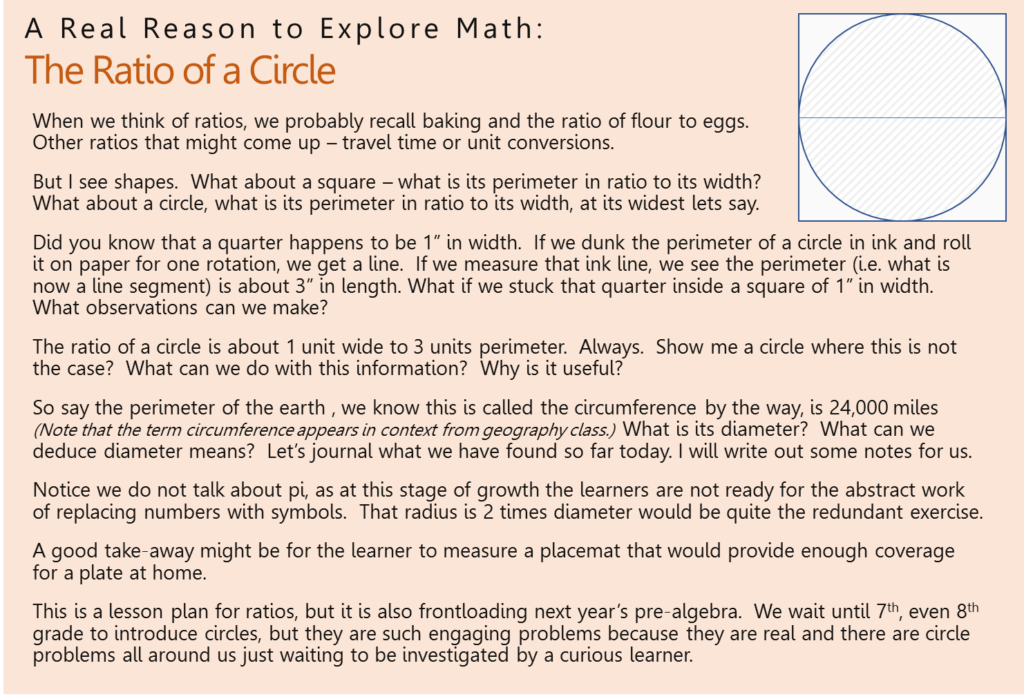
Figure 6: A Real Reason to Explore Math: The Ratio of a Circle
There are essentials to math, and the practical and the experiential must be kept in balance.
I am an essentialist to boot, believing the practical and experiential must be in balance. While Pythagoras might have professed that the step onward was all that matters, his history shows him picking up and moving to where he would be salaried to meet his basic needs. Essentialism is important, for we cannot lessen what is needed; skipped sections will impede the student down the road. Lack of ability for mental math and number sense will lead to harder work for the child as the problems grow in difficulty. Regular practice is essential to develop fluidity, stickiness and to see all the outliers. Like a music instrument, math is learned deeper if what is taught is regularly practiced.
Progressivism is a good philosophy to teach with; learners need to be at the center for math education to fully work.
My perennial and essential roots are obscured from the learner, and in the classroom, I am the facilitator with strong progressive beliefs. Progressivism is “The best way to equip [learners] with problem-solving strategies that will enable them to discover meaningful knowledge at various stages of their lives.” (Parkay, p. 127) The learner is active. I am the expert but not the answer key. The true strength in our classroom lies in the collaboration of our learners. I bring in 3-5 problems each day and the learners interests drive which to investigate. The problems are real, are at differentiated levels, and each problem targets different audience interests in the classroom.
I believe it is important to be aware of the learners’ needs in relation to cognitive areas and their stage of growth. “to think about achieving a particular goal and manage their mental processes. This is known as metacognition, and research shows that metacognitive skills help students become better problem solvers.” (Mills, 2017) It is from the learners’ actions and conversations, their investigations and misunderstandings, that I begin to surface a good set of multi-modal and differentiated paths for the classroom to travel down.