Learners are best served when they can explore a real problem, and then begin to understand the math concept.
What we want is an approach to learning that promotes student interest and develops analytical skills. I believe that learners become most interested in math when they get a chance to play with a problem before learning the math that goes into solving it. The problems that are all around us can usually be solved using different orders of math – methods picked up at different stages of our education.
A real problem can be presented, and students can try to solve it. Most learners will employ a lesser-order method to solve the problem and see the hard work that goes into doing this. Often what happens is through investigation, a few of the learners arrive at an understanding of the method in the lesson plan, before it is actually presented (see Figure 1.) In turn, they share their findings with the class.
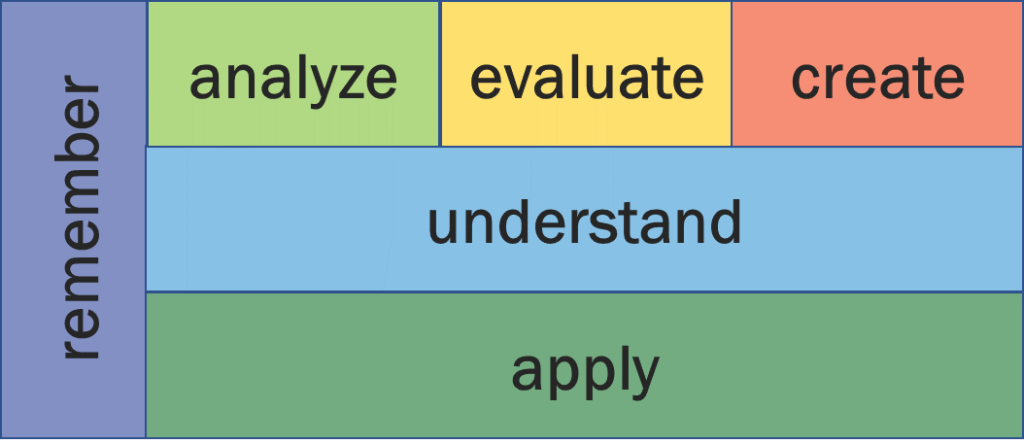
This may seem counterintuitive if you work in education services, and are familiar with Bloom’s Taxonomy of Learning (“Bloom’s Taxonomy”, 2018), as he proscribes that we first define the basic facts, explain the concepts and then have the learner use the information in new situations. What we facilitators truly want is a methodology that promotes student interest and the development of analytical skills, and putting the problem first does that.
The learner enjoys a fair struggle and is motivated to avoid hard work.
In Glenn Ellison’s “Hard Math for Elementary School” (Ellison, 2013), it is said that “hard fits well with fun.” Learners become engaged when they learn to do things that they know are hard. Math classrooms are often just not hard enough and students start to find math boring. That is why I am a strong believer in math competitions and the problems that come from these institutions. It’s not that every child will want to or should compete, it’s the engaging problems that were developed as a result of these competitions that should be incorporated into the classroom learning environment; they present a struggle, they incorporate common core math, and they can be solved using different orders of math.
And that gets to the second point of my belief – that learners are motivated to avoid hard work. When a learner solves a problem using a lower-order method, it is actually harder on them, it takes longer and more errors arise in the process. By nature, learners want to avoid hard work and this motivates them to understand the higher order methods and Mental Math.

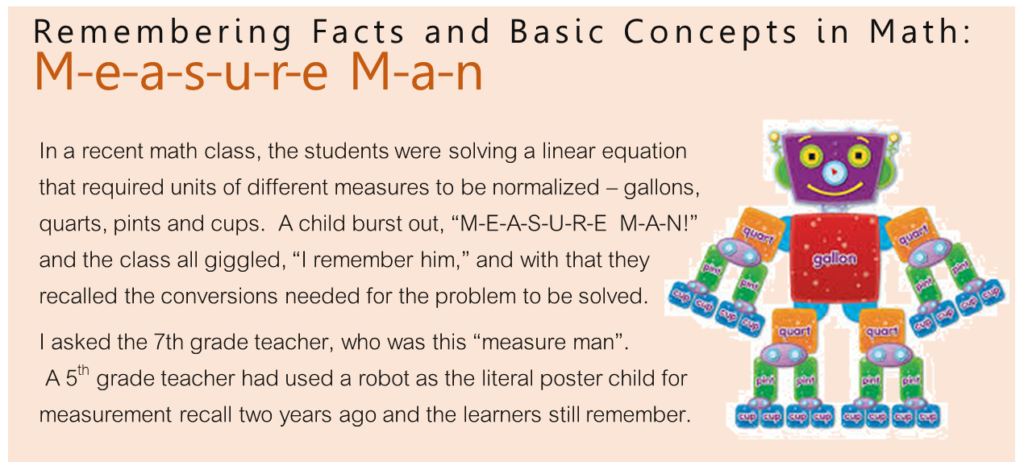
Remembering facts and basic concepts is ever-present.
I believe that every day time must be spent in math providing opportunities for learners to remember what they have successfully learned. Multi-modal approaches should be used to connect the information and help it stick.
These approaches include Do Nows, Jeopardy contests, Quizlets, and video theater, as well as rote work. The goal is to not do work for the sake of making math boring, but to give the learner the opportunity to see math in at least 3 modes and to investigate as many outliers as possible.